Integration of a fractional-rational function.
Uncertain coefficient method

We continue to work on integrating fractions. We have already looked at integrals of some types of fractions in the lesson, and this lesson, in a sense, can be considered a continuation. To successfully understand the material, basic integration skills are required, so if you have just started studying integrals, that is, you are a beginner, then you need to start with the article Indefinite integral. Examples of solutions.

Oddly enough, now we will be engaged not so much in finding integrals, but... in solving systems linear equations. In this regard urgently I recommend attending the lesson. Namely, you need to be well versed in substitution methods (“the school” method and the method of term-by-term addition (subtraction) of system equations).

What is a fractional rational function? In simple words, a fractional-rational function is a fraction whose numerator and denominator contain polynomials or products of polynomials. Moreover, the fractions are more sophisticated than those discussed in the article Integrating Some Fractions.

Integrating a Proper Fractional-Rational Function

Immediately an example and a typical algorithm for solving the integral of a fractional-rational function.

Example 1

Step 1. The first thing we ALWAYS do when solving an integral of a fractional rational function is to clarify the following question: is the fraction proper? This step is performed verbally, and now I will explain how:

First we look at the numerator and find out senior degree polynomial:

The leading power of the numerator is two.

Now we look at the denominator and find out senior degree denominator. The obvious way is to open the brackets and bring similar terms, but you can do it easier, in each find the highest degree in brackets

and mentally multiply: - thus, the highest degree of the denominator is equal to three. It is quite obvious that if we actually open the brackets, we will not get a degree greater than three.

Conclusion: Major degree of numerator STRICTLY is less than the highest power of the denominator, which means the fraction is proper.

If in this example the numerator contained the polynomial 3, 4, 5, etc. degrees, then the fraction would be wrong.

Now we will consider only the correct fractional rational functions. The case when the degree of the numerator is greater than or equal to the degree of the denominator will be discussed at the end of the lesson.

Step 2. Let's factorize the denominator. Let's look at our denominator:

Generally speaking, this is already a product of factors, but, nevertheless, we ask ourselves: is it possible to expand something else? The object of torture will undoubtedly be the square trinomial. Let's decide quadratic equation:

The discriminant is greater than zero, which means that the trinomial really can be factorized:

General rule: EVERYTHING in the denominator CAN be factored - factored

Let's begin to formulate a solution:

Step 3. Using the method of indefinite coefficients, we expand the integrand into a sum of simple (elementary) fractions. Now it will be clearer.

Let's look at our integrand function:

And, you know, somehow an intuitive thought pops up that it would be nice to turn our large fraction into several small ones. For example, like this:

The question arises, is it even possible to do this? Let us breathe a sigh of relief, the corresponding theorem of mathematical analysis states – IT IS POSSIBLE. Such a decomposition exists and is unique.

There's just one catch, the odds are Bye We don’t know, hence the name – the method of indefinite coefficients.

As you guessed, subsequent body movements are like that, don’t cackle! will be aimed at just RECOGNIZING them - to find out what they are equal to.

Be careful, I will explain in detail only once!

So, let's start dancing from:

On the left side we reduce the expression to a common denominator:

Now we can safely get rid of the denominators (since they are the same):

On the left side we open the brackets, but do not touch the unknown coefficients for now:

At the same time we repeat school rule multiplying polynomials. When I was a teacher, I learned to pronounce this rule with a straight face: In order to multiply a polynomial by a polynomial, you need to multiply each term of one polynomial by each term of the other polynomial.

From the point of view of a clear explanation, it is better to put the coefficients in brackets (although I personally never do this in order to save time):

We compose a system of linear equations.
First we look for senior degrees:

And we write the corresponding coefficients into the first equation of the system:

Remember the following point well. What would happen if there were no s on the right side at all? Let's say, would it just show off without any square? In this case, in the equation of the system it would be necessary to put a zero on the right: . Why zero? But because on the right side you can always assign this same square with zero: If on the right side there are no variables and/or a free term, then we put zeros on the right sides of the corresponding equations of the system.

We write the corresponding coefficients into the second equation of the system:

And finally, mineral water, we select free members.

Eh...I was kind of joking. Jokes aside - mathematics is a serious science. In our institute group, no one laughed when the assistant professor said that she would scatter the terms along the number line and choose the largest ones. Let's get serious. Although... whoever lives to see the end of this lesson will still smile quietly.

The system is ready:

We solve the system:

(1) From the first equation we express and substitute it into the 2nd and 3rd equations of the system. In fact, it was possible to express (or another letter) from another equation, but in this case it is advantageous to express it from the 1st equation, since there the smallest odds.

(2) We present similar terms in the 2nd and 3rd equations.

(3) We add the 2nd and 3rd equations term by term, obtaining the equality , from which it follows that

(4) We substitute into the second (or third) equation, from where we find that

(5) Substitute and into the first equation, obtaining .

If you have any difficulties with the methods of solving the system, practice them in class. How to solve a system of linear equations?

After solving the system, it is always useful to check - substitute the found values every equation of the system, as a result everything should “converge”.

Almost there. The coefficients were found, and:

The finished job should look something like this:

As you can see, the main difficulty of the task was to compose (correctly!) and solve (correctly!) a system of linear equations. And at the final stage, everything is not so difficult: we use the linearity properties of the indefinite integral and integrate. Please note that under each of the three integrals we have a “free” complex function; I talked about the features of its integration in the lesson Variable change method in indefinite integral.

Check: Differentiate the answer:

The original integrand function has been obtained, which means that the integral has been found correctly.
During the verification, we had to reduce the expression to a common denominator, and this is not accidental. The method of indefinite coefficients and reducing an expression to a common denominator are mutually inverse actions.

Example 2

Find indefinite integral.

Let's return to the fraction from the first example: . It is easy to notice that in the denominator all the factors are DIFFERENT. The question arises, what to do if, for example, the following fraction is given: ? Here we have degrees in the denominator, or, mathematically, multiples. In addition, there is a quadratic trinomial that cannot be factorized (it is easy to verify that the discriminant of the equation is negative, so the trinomial cannot be factorized). What to do? The expansion into a sum of elementary fractions will look something like with unknown coefficients at the top or something else?

Example 3

Introduce a function

Step 1. Checking if we have a proper fraction
Major numerator: 2
Highest degree of denominator: 8
, which means the fraction is correct.

Step 2. Is it possible to factor something in the denominator? Obviously not, everything is already laid out. The square trinomial cannot be expanded into a product for the reasons stated above. Hood. Less work.

Step 3. Let's imagine fractional rational function as a sum of elementary fractions.
In this case, the expansion has the following form:

Let's look at our denominator:
When decomposing a fractional-rational function into a sum of elementary fractions, three fundamental points can be distinguished:

1) If the denominator contains a “lonely” factor to the first power (in our case), then we put an indefinite coefficient at the top (in our case). Examples No. 1, 2 consisted only of such “lonely” factors.

2) If the denominator has multiple multiplier, then you need to decompose it like this:
- that is, sequentially go through all the degrees of “X” from the first to the nth degree. In our example there are two multiple factors: and , take another look at the expansion I gave and make sure that they are expanded exactly according to this rule.

3) If the denominator contains an indecomposable polynomial of the second degree (in our case), then when decomposing in the numerator you need to write linear function with uncertain coefficients (in our case with uncertain coefficients and ).

In fact, there is another 4th case, but I will keep silent about it, since in practice it is extremely rare.

Example 4

Introduce a function as a sum of elementary fractions with unknown coefficients.

This is an example for independent decision. Full solution and answer at the end of the lesson.
Follow the algorithm strictly!

If you understand the principles by which you need to expand a fractional-rational function into a sum, you can chew through almost any integral of the type under consideration.

Example 5

Find the indefinite integral.

Step 1. Obviously the fraction is correct:

Step 2. Is it possible to factor something in the denominator? Can. Here is the sum of cubes . Factor the denominator using the abbreviated multiplication formula

Step 3. Using the method of indefinite coefficients, we expand the integrand into a sum of elementary fractions:

Please note that the polynomial cannot be factorized (check that the discriminant is negative), so at the top we put a linear function with unknown coefficients, and not just one letter.

We bring the fraction to a common denominator:

Let's compose and solve the system:

(1) We express from the first equation and substitute it into the second equation of the system (this is the most rational way).

(2) We present similar terms in the second equation.

(3) We add the second and third equations of the system term by term.

All further calculations are, in principle, oral, since the system is simple.

(1) We write down the sum of fractions in accordance with the found coefficients.

(2) We use the linearity properties of the indefinite integral. What happened in the second integral? You can familiarize yourself with this method in the last paragraph of the lesson. Integrating Some Fractions.

(3) Once again we use the properties of linearity. In the third integral we begin to isolate the complete square (penultimate paragraph of the lesson Integrating Some Fractions).

(4) We take the second integral, in the third we select the complete square.

(5) Take the third integral. Ready.

TOPIC: Integration of rational fractions.

Attention! When studying one of the basic methods of integration: the integration of rational fractions, it is required to consider polynomials in the complex domain to carry out rigorous proofs. Therefore it is necessary study in advance some properties complex numbers and operations on them.

Integration of simple rational fractions.

If P(z) And Q(z) are polynomials in the complex domain, then they are rational fractions. It's called correct, if degree P(z) less degree Q(z) , And wrong, if degree R no less than a degree Q.

Any improper fraction can be represented as: ,

P(z) = Q(z) S(z) + R(z),

a R(z) – polynomial whose degree is less than the degree Q(z).

Thus, the integration of rational fractions comes down to the integration of polynomials, that is, power functions, and proper fractions, since it is a proper fraction.

Definition 5. The simplest (or elementary) fractions are the following types of fractions:

1) , 2) , 3) , 4) .

Let's find out how they integrate.

3) (studied previously).

Theorem 5. Every proper fraction can be represented as a sum of simple fractions (without proof).

Corollary 1. If is a proper rational fraction, and if among the roots of the polynomial there are only simple real roots, then in the decomposition of the fraction into the sum of simple fractions there will be only simple fractions of the 1st type:

Example 1.

Corollary 2. If is a proper rational fraction, and if among the roots of the polynomial there are only multiple real roots, then in the decomposition of the fraction into the sum of simple fractions there will be only simple fractions of the 1st and 2nd types:

Example 2.

Corollary 3. If is a proper rational fraction, and if among the roots of the polynomial there are only simple complex conjugate roots, then in the decomposition of the fraction into the sum of simple fractions there will be only simple fractions of the 3rd type:

Example 3.

Corollary 4. If is a proper rational fraction, and if among the roots of the polynomial there are only multiple complex conjugate roots, then in the decomposition of the fraction into the sum of simple fractions there will be only simple fractions of the 3rd and 4th types:

To determine the unknown coefficients in the given expansions proceed as follows. The left and right sides of the expansion containing unknown coefficients are multiplied by The equality of two polynomials is obtained. From it, equations for the required coefficients are obtained using:

1. equality is true for any values ​​of X (partial value method). In this case, any number of equations are obtained, any m of which allow one to find the unknown coefficients.

2. the coefficients coincide for the same degrees of X (method of indefinite coefficients). In this case, a system of m - equations with m - unknowns is obtained, from which the unknown coefficients are found.

3. combined method.

Example 5. Expand a fraction to the simplest.

Solution:

Let's find the coefficients A and B.

Method 1 - private value method:

Method 2 – method of undetermined coefficients:

Answer:

Integrating rational fractions.

Theorem 6. The indefinite integral of any rational fraction on any interval on which its denominator is not equal to zero exists and is expressed through elementary functions, namely rational fractions, logarithms and arctangents.

Proof.

Let's imagine a rational fraction in the form: . In this case, the last term is a proper fraction, and according to Theorem 5 it can be represented as a linear combination of simple fractions. Thus, the integration of a rational fraction is reduced to the integration of a polynomial S(x) and simple fractions, the antiderivatives of which, as has been shown, have the form indicated in the theorem.

Comment. The main difficulty in this case is the factorization of the denominator, that is, the search for all its roots.

Example 1. Find the integral

The integrand is a proper rational fraction. The expansion of the denominator into irreducible factors has the form This means that the expansion of the integrand into a sum of simple fractions has the following form:

Let's find the expansion coefficients using a combined method:

Thus,

Example 2. Find the integral

The integrand is an improper fraction, so we isolate the whole part:

The first of the integrals is tabular, and we calculate the second by decomposing the proper fraction into simple ones:

Using the method of undetermined coefficients, we have:

Thus,

Enter the function for which you need to find the integral

After calculating the indefinite integral, you will be able to receive a free DETAILED solution to the integral you entered.

Let's find the solution to the indefinite integral of the function f(x) (the antiderivative of the function).

Examples

Using degree
(square and cube) and fractions

(x^2 - 1)/(x^3 + 1)

Square root

Sqrt(x)/(x + 1)

Cube root

Cbrt(x)/(3*x + 2)

Using sine and cosine

2*sin(x)*cos(x)

arcsine

X*arcsin(x)

arc cosine

X*arccos(x)

Application of the logarithm

X*log(x, 10)

Natural logarithm

Exhibitor

Tg(x)*sin(x)

Cotangent

Ctg(x)*cos(x)

Irrational fractions

(sqrt(x) - 1)/sqrt(x^2 - x - 1)

Arctangent

X*arctg(x)

Arccotangent

X*arсctg(x)

Hyperbolic sine and cosine

2*sh(x)*ch(x)

Hyperbolic tangent and cotangent

Ctgh(x)/tgh(x)

Hyperbolic arcsine and arccosine

X^2*arcsinh(x)*arccosh(x)

Hyberbolic arctangent and arccotangent

X^2*arctgh(x)*arcctgh(x)

Rules for entering expressions and functions

Expressions can consist of functions (notations are given in alphabetical order): absolute(x) Absolute value x
(module x or |x|) arccos(x) Function - arc cosine of x arccosh(x) Arc cosine hyperbolic from x arcsin(x) Arcsine from x arcsinh(x) Arcsine hyperbolic from x arctan(x) Function - arctangent of x arctgh(x) Arctangent hyperbolic from x e e a number that is approximately equal to 2.7 exp(x) Function - exponent of x(which is e^x) log(x) or ln(x) Natural logarithm of x
(To get log7(x), you need to enter log(x)/log(7) (or, for example, for log10(x)=log(x)/log(10)) pi The number is "Pi", which is approximately equal to 3.14 sin(x) Function - Sine of x cos(x) Function - Cosine of x sinh(x) Function - Sine hyperbolic from x cosh(x) Function - Cosine hyperbolic from x sqrt(x) Function - square root of x sqr(x) or x^2 Function - Square x tan(x) Function - Tangent from x tgh(x) Function - Tangent hyperbolic from x cbrt(x) Function - cube root of x

The following operations can be used in expressions: Real numbers enter as 7.5 , Not 7,5 2*x- multiplication 3/x- division x^3- exponentiation x+7- addition x - 6- subtraction
Other features: floor(x) Function - rounding x downward (example floor(4.5)==4.0) ceiling(x) Function - rounding x upward (example ceiling(4.5)==5.0) sign(x) Function - Sign x erf(x) Error function (or probability integral) laplace(x) Laplace function

The problem of finding the indefinite integral of a fractionally rational function comes down to integrating simple fractions. Therefore, we recommend that you first familiarize yourself with the section of the theory of decomposition of fractions into the simplest.

Example.

Solution.

Since the degree of the numerator of the integrand is equal to the degree of the denominator, we first select the whole part by dividing the polynomial by the polynomial with a column:

That's why, .

The decomposition of the resulting proper rational fraction into simpler fractions has the form . Hence,

The resulting integral is the integral of the simplest fraction of the third type. Looking ahead a little, we note that you can take it by subsuming it under the differential sign.

Because , That . That's why

Hence,

Now let's move on to describing methods for integrating simple fractions of each of the four types.

Integration of simple fractions of the first type

The direct integration method is ideal for solving this problem:

Example.

Solution.

Let's find the indefinite integral using the properties of the antiderivative, the table of antiderivatives and the integration rule.

Top of page

Integration of simple fractions of the second type

The direct integration method is also suitable for solving this problem:

Example.

Solution.

Top of page

Integration of simple fractions of the third type

First we present the indefinite integral as a sum:

We take the first integral by subsuming it under the differential sign:

That's why,

Let us transform the denominator of the resulting integral:

Hence,

The formula for integrating simple fractions of the third type takes the form:

Example.

Find the indefinite integral .

Solution.

We use the resulting formula:

If we didn’t have this formula, what would we do:

9. Integration of simple fractions of the fourth type

The first step is to put it under the differential sign:

The second step is to find an integral of the form . Integrals of this type are found using recurrence formulas. (See partitioning using recurrence formulas). The following recurrent formula is suitable for our case:

Example.

Find the indefinite integral

Solution.

For this type of integrand we use the substitution method. Let's introduce a new variable (see the section on integration of irrational functions):

After substitution we have:

We came to finding the integral of a fraction of the fourth type. In our case we have coefficients M = 0, p = 0, q = 1, N = 1 And n=3. We apply the recurrent formula:

After reverse replacement we get the result:

10. Integration of trigonometric functions.

Many problems come down to finding integrals of transcendental functions containing trigonometric functions. In this article we will group the most common types of integrands and use examples to consider methods for their integration.

Let's start by integrating sine, cosine, tangent and cotangent.

From the table of antiderivatives we immediately note that And .

The method of subsuming the differential sign allows you to calculate the indefinite integrals of the tangent and cotangent functions:

Top of page

Let's look at the first case, the second is absolutely similar.

Let's use the substitution method:

We came to the problem of integrating an irrational function. The substitution method will also help us here:

All that remains is to carry out the reverse replacement and t = sinx:

Top of page

You can learn more about the principles of finding them in the section integration using recurrent formulas. If you study the derivation of these formulas, you can easily take integrals of the form , Where m And n– natural numbers.

Top of page

Top of page

The most creativity comes when the integrand contains trigonometric functions with different arguments.

This is where the basic formulas of trigonometry come to the rescue. So write them down on a separate piece of paper and keep them before your eyes.

Example.

Find the set of antiderivatives of a function .

Solution.

The reduction formulas give And .

That's why

The denominator is the formula for the sine of the sum, therefore,

We arrive at the sum of three integrals.

Top of page

Integrands containing trigonometric functions can sometimes be reduced to fractional rational expressions using standard trigonometric substitution.

Let's write out trigonometric formulas expressing sine, cosine, tangent through the tangent of the half argument:

When integrating, we also need the differential expression dx through the tangent of the half angle.

Because , That

That is, , where.

Example.

Find the indefinite integral .

Solution.

Let's use standard trigonometric substitution:

Thus, .

Decomposing the integrand into simple fractions leads us to the sum of two integrals:

All that remains is to carry out the reverse replacement:

11. Recurrence formulas are formulas that express n The th member of the sequence through the previous members. They are often used when finding integrals.

We do not aim to list all recurrent formulas, but want to give the principle of their derivation. The derivation of these formulas is based on the transformation of the integrand and the application of the method of integration by parts.

For example, the indefinite integral can be taken using the recurrence formula .

Derivation of the formula:

Using trigonometry formulas, we can write:

We find the resulting integral by the method of integration by parts. As a function u(x) let's take cosx, hence, .

That's why,

We return to the original integral:

That is,

That's what needed to be shown.

The following recurrence formulas are derived similarly:

Example.

Find the indefinite integral.

Solution.

We use the recurrent formula from the fourth paragraph (in our example n=3):

Since from the table of antiderivatives we have , That

“A mathematician, just like an artist or poet, creates patterns. And if his patterns are more stable, it is only because they are composed of ideas... The patterns of a mathematician, just like the patterns of an artist or a poet, must be beautiful; Ideas, just like colors or words, must correspond to each other. Beauty is the first requirement: there is no place in the world for ugly mathematics».

G.H.Hardy

In the first chapter it was noted that there are primitives quite simple functions, which can no longer be expressed through elementary functions. In this regard, those classes of functions about which we can accurately say that their antiderivatives are elementary functions acquire enormous practical importance. This class of functions includes rational functions, representing the ratio of two algebraic polynomials. Many problems lead to the integration of rational fractions. Therefore, it is very important to be able to integrate such functions.

2.1.1. Fractional rational functions

Rational fraction(or fractional rational function) is the relation of two algebraic polynomials:

where and are polynomials.

Let us remind you that polynomial (polynomial, whole rational function) nth degree called a function of the form

Where – real numbers. For example,

– polynomial of the first degree;

– polynomial of the fourth degree, etc.

The rational fraction (2.1.1) is called correct, if the degree is lower than the degree , i.e. n<m, otherwise the fraction is called wrong.

Any improper fraction can be represented as the sum of a polynomial (the whole part) and a proper fraction (the fractional part). The separation of the whole and fractional parts of an improper fraction can be done according to the rule for dividing polynomials with a “corner”.

Example 2.1.1. Identify the whole and fractional parts of the following improper rational fractions:

A) , b) .

Solution . a) Using the “corner” division algorithm, we get

Thus, we get

.

b) Here we also use the “corner” division algorithm:

As a result, we get

.

Let's summarize. In the general case, the indefinite integral of a rational fraction can be represented as the sum of the integrals of the polynomial and the proper rational fraction. Finding antiderivatives of polynomials is not difficult. Therefore, in what follows we will mainly consider proper rational fractions.

2.1.2. The simplest rational fractions and their integration

Among proper rational fractions, there are four types, which are classified as the simplest (elementary) rational fractions:

3) ,

4) ,

where is an integer, , i.e. quadratic trinomial has no real roots.

Integrating simple fractions of type 1 and type 2 does not present much difficulty:

, (2.1.3)

. (2.1.4)

Let us now consider the integration of simple fractions of the 3rd type, but we will not consider fractions of the 4th type.

Let's start with integrals of the form

.

This integral is usually calculated by isolating the perfect square of the denominator. The result is a table integral of the following form

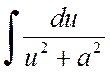or .

Example 2.1.2. Find the integrals:

A) 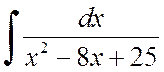, b) .

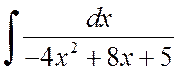Solution . a) Select a complete square from a quadratic trinomial:

From here we find

b) By isolating a complete square from a quadratic trinomial, we obtain:

Thus,

.

To find the integral

you can isolate the derivative of the denominator in the numerator and expand the integral into the sum of two integrals: the first of them by substitution comes down to appearance

,

and the second - to the one discussed above.

Example 2.1.3. Find the integrals:

.

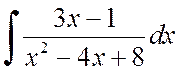Solution . Note that 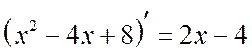. Let us isolate the derivative of the denominator in the numerator:

The first integral is calculated using substitution :

In the second integral, we select the perfect square in the denominator

Finally, we get

2.1.3. Proper rational fraction expansion
for the sum of simple fractions

Any proper rational fraction can be represented in a unique way as a sum of simple fractions. To do this, the denominator must be factorized. From higher algebra it is known that every polynomial with real coefficients